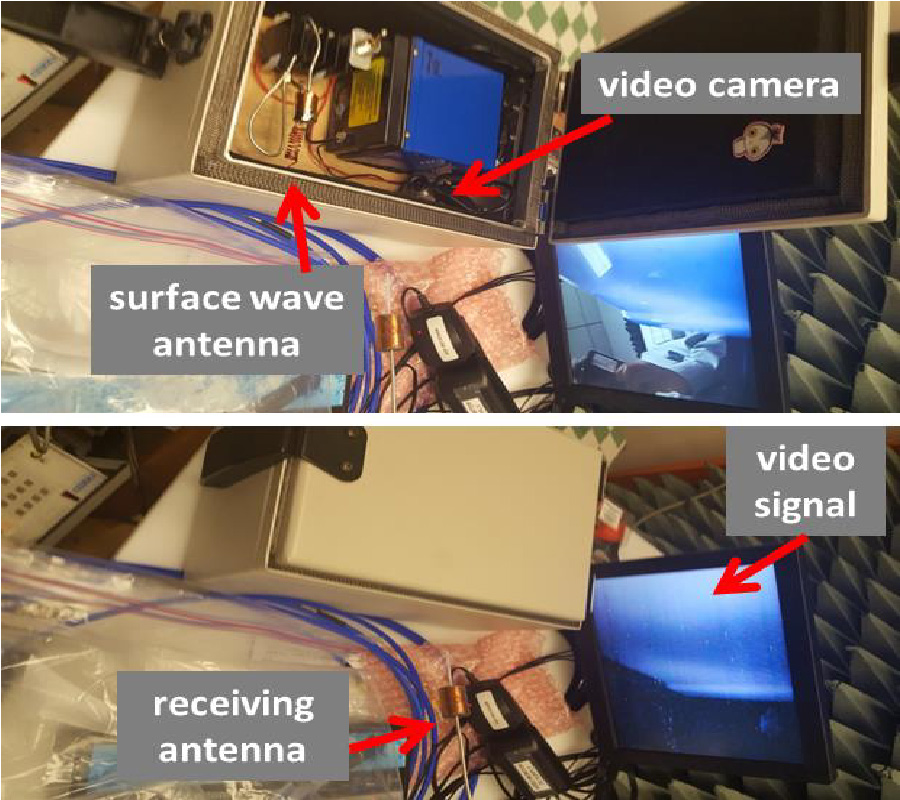
Scatterer Characterization Based on the Condiagonalization of the Sinclair Backscattering Matrix
Georgios Kouroupis and
Vassilis Anastassopoulos
In this paper we revisit the condiagonalization of the Sinclair backscattering matrix, to overcome the Huynen decomposition issues, so as to correctly extract scatterer polarimetric properties. The correct extraction of scatterer polarimetric properties will lead to the correct classification of the scatterer predominant scattering mechanism. Huynen used the congruence transformation by a special unitary matrix to diagonalize the Sinclair matrix into a real and nonnegative diagonal matrix. He also expressed the special unitary matrix in terms of the polarization ellipse parameters and associated them with the scatterer orientation, asymmetry, and skip angle. Unfortunately, this association was found misleading. As a result, it makes the scatterer classification ambiguous, for it is based on the scatterer skip angle and the diagonal matrix. To overcome these ambiguities, we perform the diagonalization procedure founded on the consimilarity transformation by a special unitary matrix, as proposed by Lüneberg. In order to correctly extract the scatterer asymmetry degree and orientation, we express the special unitary matrix in terms of an asymmetry operation and a pure rotation operation. Moreover, we integrate the scatterer skip angle in the diagonal matrix of the consimilarity transformation by having it complex, leading to an unequivocal scatterer characterization.