PIER B | PIER Journals

2010-03-02

PIER B

Vol. 19, 445-473, 2010

download: 249

A Domain Decomposition Method Based on a Generalized Scattering Matrix Formalism and a Complex Source Expansion

Enrica Martini , Giacomo Carli and Stefano Maci

A general domain decomposition scheme based on the use of complex sources is presented for the electromagnetic analysis of complex antenna and/or scattering problems. The analysis domain is decomposed into separate subdomains whose interactions are described through a network formalism, where the ports are associated with complex point source (CPS) beams radially emerging from the subdomain boundaries. Each obstacle is independently analyzed with the most appropriate technique and described through a generalized scattering matrix (GSM). Finally, a linear system is constructed, where the excitation vector is given by the complex source expansion of the primary sources. Thanks to the angular selectivity of the CPS beams, the subdomain interactions only involve a small fraction of the beams; thus, yielding sparse moderate size linear systems. Due to the re-usability of the GSMs, the proposed approach is particularly efficient in the context of parametric studies or antenna installation problems. Numerical examples are provided to demonstrate the efficiency and the accuracy of the proposed strategy.

A DOMAIN DECOMPOSITION METHOD BASED ON A GENERALIZED SCATTERING MATRIX FORMALISM AND A COMPLEX SOURCE EXPANSION

2010-02-26

PIER B

Vol. 19, 427-444, 2010

doi:10.2528/PIERB09123003

download: 385

Dielectric Resonator Antenna Mounted on a Circular Cylindrical Ground Plane

Saber Zainud-Deen , Hend Abd El-Azem Malhat and Kamal Awadalla

In this paper, the radiation characteristics of the single- element cylindrical dielectric resonator antenna mounted on the surface of a metallic hollow circular cylindrical structure is investigated. The effect of the radius of curvature on the return loss, input impedance, standing wave ratio, and radiation pattern is explored. Mutual coupling between two identical cylindrical dielectric resonator antennas on a cylindrical structure in different configurations is determined. To reduce the mutual coupling between the two antennas, the surface of the cylinderical ground plane is defected by cutting slots, or inserting quarter wavelength grooves between the two antennas. The finite element method and the finite integration technique are used to calculate the radiation characteristics of the antenna.

DIELECTRIC RESONATOR ANTENNA MOUNTED ON A CIRCULAR CYLINDRICAL GROUND PLANE

2010-02-22

PIER B

Vol. 19, 405-425, 2010

doi:10.2528/PIERB09112309

download: 254

Improved Cfo Algorithm for Antenna Optimization

Richard Formato

An improved Central Force Optimization (CFO) algorithm for antenna optimization is presented. CFO locates the global extrema an objective function to be maximized, in this case antenna directivity, by flying "probes" through the decision space (DS). The new implementation includes variable initial probe distribution and decision space adaptation. CFO's performance is assessed against a recognized antenna benchmark problem specifically designed to evaluate optimization evolutionary algorithms for antenna applications. In addition, summary results also are presented for a standard twenty-three function suite of analytic benchmarks. The improved CFO implementation exhibits excellent performance.

IMPROVED CFO ALGORITHM FOR ANTENNA OPTIMIZATION

2010-02-16

PIER B

Vol. 19, 385-403, 2010

doi:10.2528/PIERB09122201

download: 222

Effects of Geometry on Amplification Property of Erbium Doped Holey Fiber Amplifiers Using Scalar Effective Index Method

Maryam Karimi and Faramarz E. Seraji

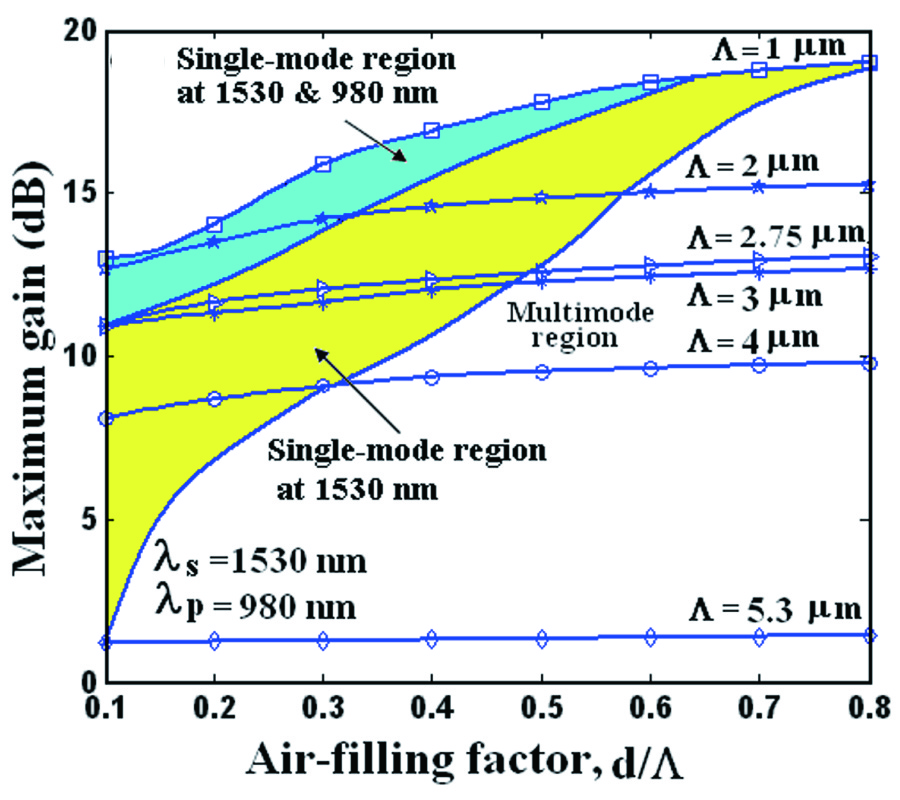
Holey fibers (HF) with their peculiar properties have been used in fabrication of Erbium doped holey fiber amplifiers (EDHFA) for third optical communication window. In this paper, by using scalar effective index method, the analyses are presented to investigate the effects of HF geometrical parameters on the gain performance of the EDHFAs. The hierarchical variations of the parameters, including the air-hole sizes (AHS), propagating modes of the core and cladding, mode field diameter of the signal and pump, would cause alterations in the maximum gain and the optimum lengths of the EDHFAs. By determining the normalized frequency of the HF in wide range variations of the air-hole diameter, air-hole spacing, and air-filling factor (AFF), the single-mode regions for signal and pump wavelengths are obtained, where the maximum gain and the optimum lengths are evaluated. In addition, the influence of pump power and the dopant concentration in terms of the AFF are investigated. It is shown that by using suitable AHS and AFF, one can obtain a higher gain for a shorter optimum length in the EDHFAs. The obtained results can be a useful tool for design of HF-based optical amplifiers with lesser effects of amplified spontaneous emission and nonlinearities because of shorter optimized length.

EFFECTS OF GEOMETRY ON AMPLIFICATION PROPERTY OF ERBIUM DOPED HOLEY FIBER AMPLIFIERS USING SCALAR EFFECTIVE INDEX METHOD

2010-02-04

PIER B

Vol. 19, 367-383, 2010

doi:10.2528/PIERB09122102

download: 290

Minimum Usage of Ferrite Tiles in Anechoic Chambers

Sayed Razavi , Mohammad Khalaj-Amirhosseini and Ahmad Cheldavi

Anechoic chambers which are used for emission and immunity testing require expensive ferrite tiles on their inner surfaces. This paper describes a method to reduce the number of required ferrite tiles, whilst ensuring a reliable and specified test region. In this method, the positions of some ferrite tiles are found optimally to keep the performance of the anechoic chamber as high as possible. An optimum ray-tracing method is presented to predict the electric field in the anechoic chamber. The performance of the proposed method is verified by a comprehensive example simulated by the CST software, which is a full-wave simulator based on time difference method.

MINIMUM USAGE OF FERRITE TILES IN ANECHOIC CHAMBERS

2010-01-27

PIER B

Vol. 19, 329-366, 2010

doi:10.2528/PIERB09110502

download: 194

Weakly Conditionally Stable and Unconditionally Stable FDTD Schemes for 3D Maxwell's Equations

Juan Chen and Jianguo Wang

To overcome the Courant limit on the time step size of the conventional finite-difference time-domain (FDTD) method, some weakly conditionally stable and unconditionally stable FDTD methods have been developed recently. To analyze the relations between these methods theoretically, they are all viewed as approximations of the conventional FDTD scheme in present discussion. The errors between these methods and the conventional FDTD method are presented analytically, and the numerical performances, including computation accuracy, efficiency, and memory requirements, are discussed, by comparing with those of the conventional FDTD method.

WEAKLY CONDITIONALLY STABLE AND UNCONDITIONALLY STABLE FDTD SCHEMES FOR 3D MAXWELL'S EQUATIONS

2010-01-27

PIER B

Vol. 19, 305-327, 2010

doi:10.2528/PIERB09121609

download: 399

Planar Antennas for Passive UHF RFID Tag

Arun Kumar , Davinder Parkash and Machavaram Kartikeyan

In this paper, design, fabrication, and testing of Radio Frequency Identification (RFID) antennas for the European Telecommunications Standards Institute (ETSI) and Federal Communications Commission (FCC) bands are discussed. The designs proposed in this paper are for UHF RFID tag that conforms to EPCglobal C1G2 1.2.0. The exceptional characteristics of the RFID are investigated in terms of antenna-IC matching and radiation efficiency. The proposed RFID antennas have been fabricated on 5 mil thick Flexible Copper Clad Laminate and the read range of the proposed RFID antennas is experimentally tested. Measured free air read range of all proposed designs is over 4 m. The performance of the tag antenna design affixed to various objects is also tested with read range measurements. The results show that the antenna designs can be used for tagging cardboard and plastic objects.

PLANAR ANTENNAS FOR PASSIVE UHF RFID TAG

2010-01-26

PIER B

Vol. 19, 285-303, 2010

doi:10.2528/PIERB09092308

download: 185

Novel Method to Analyze and Design One-Dimensional Reciprocal Periodic Structures with Symmetrical Cells

Omid Zandi , Zahra Atlasbaf and Mohammad Abrishamian

The dispersion relation is derived for the most general configuration of a passive and reciprocal periodically loaded transmission line in a unique and simple form by introducing two novel parameters. Based on this relation, the phase and group velocities are determined and a simple condition for phase reversal propagation is obtained. The two above mentioned parameters help us to develop a polar diagram to model the behavior of any two-port network as a function of frequency. By this diagram, we can determine the direction of the phase velocity and also the value of the propagation constant. Then, symmetrical cells and thereof the periodic structures composed of them are analyzed. For such structures, it will be shown that the dispersion relation can be rewritten in a form similar to the Lorentz transformation. We design and analyze a bandstop filter to verify the method.

NOVEL METHOD TO ANALYZE AND DESIGN ONE-DIMENSIONAL RECIPROCAL PERIODIC STRUCTURES WITH SYMMETRICAL CELLS

2010-01-20

PIER B

Vol. 19, 263-283, 2010

doi:10.2528/PIERB09121504

download: 204

Novel Liquid Crystal Tunable Flat-Top Optical Interleaver

Shadi A. Alboon , Alaeddin Abu-Abed , Robert Lindquist and Hussein R. Al-Zoubi

In this paper, we propose tunable optical interleaver filters based on the combined Michelson interferometer (MI) and the Gires-Tournois interferometer (GTI) with polarization diversity. The tuning capability is achieved by integrating liquid crystals into the interleaver. In addition to the tunability, it is also shown that the response for this proposed interleaver has a flat-top. Various GTIMI LC-based interleaver structures are discussed in this paper and their performance, in terms of the flat-top and the pass band ripple, are judged. These structures have the advantages of low operation voltage requirements and design simplicity. A GTI-MI interleaver has been fabricated and tested. The experimental results show that the interleaver has tunable response.

NOVEL LIQUID CRYSTAL TUNABLE FLAT-TOP OPTICAL INTERLEAVER

2010-01-19

PIER B

Vol. 19, 233-261, 2010

doi:10.2528/PIERB09120904

download: 268

Frequency Dispersion Limits Resolution in Veselago Lens

Robert Collin

The properties of a lossless Veselago lens is examined when the material parameters epsilon and mu are frequency dispersive. A complete solution is presented that is based on the use of Fourier transforms in the frequency domain and is obtained in terms of the residues at the poles and branch cut integrals. It is shown that for an incident field with a finite frequency spectrum the excited evanescent field consists of resonant even and odd surface wave modes that do not grow exponentially within the slab. For a lossless slab and a sinusoidal signal of finite duration, at a single frequency corresponding to that where the relative values of epsilon and mu equal -1, Pendry's solution is obtained along with excited surface wave modes and other interfering waves that makes it impossible to obtain a coherent reconstruction of the spatial spectrum of the object field at the image plane. If the slab material is lossy the excited interfering surface wave modes will decay away in a relatively short time interval, but as shown by other investigators the resolution of the lens will be reduced in a very substantial way if the losses are moderate to large.

FREQUENCY DISPERSION LIMITS RESOLUTION IN VESELAGO LENS

2010-01-19

PIER B

Vol. 19, 205-231, 2010

doi:10.2528/PIERB09110602

download: 257

Nonlinear Pulse Propagation in a Weakly Birefringent Optical Fiber Part 1: Derivation of Coupled Nonlinear Schrodinger Equations (Cnlse)

Natarajan Kalyanasundaram and P. Muthuchidambaranathan

A systematic derivation of the Coupled Nonlinear Schrodinger Equations (CNLSE) governing nonlinear pulse propagation in a weakly birefringent monomode optical fiber based on a multiple-scale perturbation solution of the semilinear vector wave equation for the electric field in a (randomly) birefringent fiber medium is presented. The analysis of the nonlinear propagation characteristics of optical pulses based on a numerical solution of the CNLSE is deferred to the second part of this contribution.

NONLINEAR PULSE PROPAGATION IN A WEAKLY BIREFRINGENT OPTICAL FIBER PART 1: DERIVATION OF COUPLED NONLINEAR SCHRODINGER EQUATIONS (CNLSE)

2010-01-15

PIER B

Vol. 19, 177-203, 2010

doi:10.2528/PIERB09112402

download: 321

Electromagnetic Fields in a Cavity Filled with Some Nonstationary Media

Mariya S. Antyufeyeva and Oleg Tretyakov

The paper presents an analytical approach to treat the problem of transient oscillations in a cavity uniformly filled with nonstationary medium, which is characterized by time-varying permittivity and conductivity. Closed-form solutions are found for some transient excitations and medium parameters.

ELECTROMAGNETIC FIELDS IN A CAVITY FILLED WITH SOME NONSTATIONARY MEDIA

2010-01-13

PIER B

Vol. 19, 151-176, 2010

doi:10.2528/PIERB09102606

download: 181

Mode Expansion in Time Domain for Conical Lines with Angular Medium Inhomogeneity

Alexander Butrym and Bogdan A. Kochetov

A new modification of the method of Mode Expansion in Time Domain is proposed for studying transient signals propagation in conical lines (including multi-connected ones) with inhomogeneous and time-dependent medium. The method is based on expanding the fields in spherical coordinate system into series of angular dependent modes with mode amplitudes being governed by a system of coupled evolutionary equations. The medium parameters (permittivity and permeability) are taken in a factorized form as a product of angular dependent factor and a factor that depends on time and radial coordinate. The introduced method can be applied to analysis of propagation and radiation in conical-like antennas with dielectric filling.

MODE EXPANSION IN TIME DOMAIN FOR CONICAL LINES WITH ANGULAR MEDIUM INHOMOGENEITY

2010-01-08

PIER B

Vol. 19, 133-150, 2010

doi:10.2528/PIERB09110505

download: 150

Dispersion of Electromagnetic Waves Guided by an Open Tape Helix II

Natarajan Kalyanasundaram and Gnanamoorthi Babu

The dispersion equation for electromagnetic waves guided by an open tape helix for the standard model of an infinitesimally thin and perfectly conducting tape is derived from an exact solution of a homogeneous boundary value problem for Maxwell's equations. A numerical analysis of the dispersion equation reveals that the tape current density component perpendicular to the winding direction does not affect the dispersion characteristics to any significant extent. In fact, there is a significant deviation from the dominant-mode sheath-helix dispersion curve only in the third allowed region and towards the end of the second allowed region. It may be concluded that the anisotropically conducting model of the tape helix that neglects the above transverse-current contribution is a good approximation to the isotropically conducting model that takes into account this contribution except at high frequencies even for wide tapes.

DISPERSION OF ELECTROMAGNETIC WAVES GUIDED BY AN OPEN TAPE HELIX II

2010-01-06

PIER B

Vol. 19, 115-131, 2010

doi:10.2528/PIERB09111904

download: 166

Electromagnetic Eigenmodes in Matter. Van Der Waals-London and Casimir Forces

Marian Apostol and Georgeta Vaman

We derive van der Waals-London and Casimir forces by calculating the eigenmodes of the electromagnetic field interacting with two semi-infinite bodies (two halves of space) with parallel surfaces separated by distance d. We adopt simple models for metals and dielectrics, well-known in the elementary theory of dispersion. In the non-retarded (Coulomb) limit we get a d^-3-force (van der Waals-London force), arising from the zero-point energy (vacuum fluctuations) of the surface plasmon modes. When retardation is included we obtain a d^-4-(Casimir) force, arising from the zero-point energy of the surface plasmon-polariton modes (evanescent modes) for metals, and from propagating (polaritonic) modes for identical dielectrics. The same Casimir force is also obtained for "fixed surfaces" boundary conditions, irrespective of the pair of bodies. The approach is based on the equation of motion of the polarization and the electromagnetic potentials, which lead to coupled integral equations. These equations are solved, and their relevant eigenfrequencies branches are identified.

2010-01-01

PIER B

Vol. 19, 95-114, 2010

doi:10.2528/PIERB09111105

download: 167

Equation Solution for the Current in Radial Impedance Monopole on the Perfectly Conducting Sphere

Mikhail Nesterenko , Dmitriy Yu. Penkin , Victor A. Katrich and Victor M. Dakhov

The problem about the electrical current distribution along thin radial impedance monopole, located on the perfectly conducting sphere, has been solved in a rigorous electrodynamic formulation in the paper. The problem formulation strictness is provided by the use of the Green's function for the Hertz's vector potential for unbounded space outside the perfectly conducting sphere at formulation of the initial integral equation concerning the current in monopole. The approximate analytical solution of the integral equation has been obtained by the method of iterations both for the case of excitation of the monopole by the δ-generator of voltage, located on the finite distance over the spherical scatterer, and at the excitation of the monopole at its basis.

EQUATION SOLUTION FOR THE CURRENT IN RADIAL IMPEDANCE MONOPOLE ON THE PERFECTLY CONDUCTING SPHERE

2009-12-17

PIER B

Vol. 19, 65-93, 2010

doi:10.2528/PIERB09111305

download: 175

Single-Mode Propagation of Light in One-Dimensional All-Dielectric Light-Guiding Systems

Changbiao Wang

Numerical results are presented for single-mode guidance, which is based on photonic band gap (PBG) effect, in one-dimensional planar all-dielectric light-guiding systems. In such systems there may be two kinds of light-speed point (the intersection of a mode-dispersion curve and the light line of guiding region ambient medium): One is the intrinsic light-speed point that is independent of the guiding region width, and the other is the movable light-speed point that varies with the guiding region width. It is found that the intrinsic light-speed point plays an important role to form the single-mode regime by destroying the coexistence of the lowest guided TM and TE modes that are born with a degeneration point. A mode-lost phenomenon is exposed and this phenomenon suggests a way of how to identify PBG-guided fundamental modes. Quasi-cutofffree index-guided modes in the PBG guiding structures are examined, which appear when the higher-index layers are adjacent to the guiding region and the guiding region width is small. The transverse resonance condition is derived in the Maxwell optics frame, and it is shown that there is a significant revision to the traditional one in the ray optics model. A sufficient and necessary condition for intrinsic light-speed points is given, which provides strong support to the numerical results.

SINGLE-MODE PROPAGATION OF LIGHT IN ONE-DIMENSIONAL ALL-DIELECTRIC LIGHT-GUIDING SYSTEMS

2009-12-14

PIER B

Vol. 19, 41-63, 2010

doi:10.2528/PIERB09110907

download: 1335

Degree of Roughness of Rough Layers: Extensions of the Rayleigh Roughness Criterion and Some Applications

Nicolas Pinel , Christophe Bourlier and Joseph Saillard

In the domain of electromagnetic wave propagation in the presence of rough surfaces, the Rayleigh roughness criterion is a widely-used means to estimate the degree of roughness of considered surface. In this paper, this Rayleigh roughness criterion is extended to the case of rough layers. Thus, it provides an interesting qualitative tool for estimating the degree of electromagnetic roughness of rough layers.

DEGREE OF ROUGHNESS OF ROUGH LAYERS: EXTENSIONS OF THE RAYLEIGH ROUGHNESS CRITERION AND SOME APPLICATIONS

2009-12-10

PIER B

Vol. 19, 21-40, 2010

doi:10.2528/PIERB09111106

download: 251

The Propagation Problem in a BI-Isotropic Waveguide

Andreas D. Ioannidis , Gerhard Kristensson and Daniel Sjöberg

We investigate the problem of defining propagating constants and modes in metallic waveguides of an arbitrary cross section, filled with a homogeneous bi-isotropic material. The approach follows the guidelines of the classical theory for the isotropic, homogeneous, lossless waveguide: starting with the Maxwell system, we formulate a spectral problem where the square of the propagation constant shows up as the eigenvalue and the corresponding mode as the eigenvector. The difficulty that arises, and this is a feature of chirality, is that the eigenvalue is involved in the boundary conditions. The main result is that the problem is solvable whenever the Dirichlet problem for the Helmholtz equation in the cross section is solvable and a technical hypothesis is fulfilled. Our method, inspired by the null-field method, is quite general and has a good potential to work in various geometries.