PIER M | PIER Journals

2017-07-28

PIER M

Vol. 58, 205-213, 2017

download: 307

Energy-Efficient Communication in Large Scale Antenna Systems: Impact of Variable User Capacity and Number of Transmission Antennas

Joseph Isabona and Viranjay M. Srivastava

Energy-efficient transmission is fast becoming a critical factor in designing future mobile broadband cellular communication systems. This research work examines the constraints with regard to the achievable throughput and energy efficiency that can be attained on the use of precoding-based massive MIMO systems, bearing in mind the effect of some key performance impacting parameters. We first introduced an absolute energy efficiency-based model to evaluate the deep-down relationship among the packet length, the Bit error rate (BER) and throughput. Then, by means of simulation with cyclic coordinated search algorithm, optimal achievable throughput and energy efficiency performance have been shown and demonstrated for variable capacity of users and number of transmission antennas. This work is expected to be of enormous importance to practical system design on the use of massive MIMO antenna technology for data throughput and energy efficiency maximization in future 5G systems.

ENERGY-EFFICIENT COMMUNICATION IN LARGE SCALE ANTENNA SYSTEMS: IMPACT OF VARIABLE USER CAPACITY AND NUMBER OF TRANSMISSION ANTENNAS

2017-07-24

PIER M

Vol. 58, 193-204, 2017

doi:10.2528/PIERM17022704

download: 430

Tuning of Microwave Absorption Properties and Electromagnetic Interference (EMI) Shielding Effectiveness of Nanosize Conducting Black-Silicone Rubber Composites Over 8-18 GHz

Raj Kumar Jani , Manoj Kumar Patra , Lokesh Saini , Anuj Shukla , Chandra Pal Singh and Sampat Raj Vadera

In this paper, studies on broadband microwave absorption and electromagnetic shielding effectiveness are reported in flexible rubber composites with low filler content of nanosize conducting carbon over 8-18 GHz frequency range of electromagnetic spectrum. Rubber based composites are prepared by loading of 1-15 wt% nanosize conducting Carbon Black (CB) in silicone rubber matrix. Effect of percentage loading of nanosize CB on DC conductivity, dielectric & microwave absorption properties and electromagnetic Shielding Effectiveness (SE) of silicone rubber composites is studied. The percolation threshold is achieved at low concentration (3 wt%) of CB in composites. The observed complex permittivity values revealed that composites with concentration of 5 wt% CB can provide more than 90% microwave absorption (Reflection Loss > -10 dB) over 8-18 GHz at composite thickness of 1.9-2.7 mm. Further, composites with concentration of 15 wt% of CB shows -40 dB SE over the broad frequency range 8-18 GHz at thickness 2.8 mm. The effect of composite thickness on microwave absorption properties and shielding effectiveness is also analyzed. Thus, the prepared rubber composites with suitable concentration of nanosize CB as filler may be used as microwave absorber in stealth applications as well as for EMI shielding of electronic equipments in various civilian and military areas.

TUNING OF MICROWAVE ABSORPTION PROPERTIES AND ELECTROMAGNETIC INTERFERENCE (EMI) SHIELDING EFFECTIVENESS OF NANOSIZE CONDUCTING BLACK-SILICONE RUBBER COMPOSITES OVER 8-18 GHZ

2017-07-19

PIER M

Vol. 58, 183-191, 2017

doi:10.2528/PIERM17050304

download: 309

A Virtual Space-Time Adaptive Beamforming Method for Space-Time Antijamming

Fulai Liu , Ruiyan Du and Xiaoyu Bai

Space-time antijamming problem has received significant attention recently in the passive radar systems, such as Global Navigation Satelite Systems (GNSS). These space-time beamformers use two adaptive filters, i.e., spatial filter and temporal filter for canceling interference signals. However, most of the work on spacetime antijamming problem presented in the literature require multiple antennas and delay taps. In this paper, a virtual space-time adaptive beamforming method is proposed. The temporal smoothing technique is utilized to add a structure of the received data model for the implementation of the proposed method without delay tap. Compared with the previous work, the presented method offers a number of advantages over other recently proposed algorithms. For example, the space-time weight vector can be obtained by simple algebraic operations. It has lower computational complexity.It can reduce system overhead since the temporal smoothing technology is used instead of multiple delay taps. Simulation results are presented to verify that effectiveness of the proposed method.

A VIRTUAL SPACE-TIME ADAPTIVE BEAMFORMING METHOD FOR SPACE-TIME ANTIJAMMING

2017-07-19

PIER M

Vol. 58, 171-181, 2017

doi:10.2528/PIERM17050101

download: 357

Effects of Reentry Plasma Fluctuation on Polarization Properties of Electromagnetic Waves

Xinglai Wang , Zhiwei Liu and Guojiang Xia

Fluctuations of the reentry plasma sheath can affect the propagation of Electromagnetic waves. The relations between fluctuations and the propagation of electromagnetic waves are analyzed. The effects on polarization propertiesin L-band, S-band and Ka-band during a typical reentry process are studied using methods derived by synthesizing the compressible turbulent flow theory, plasma theory, and electromagnetic wave theory together. Results show that in L-band and S-band, the effects increase with the altitude, while in Ka-band, the effects decrease with altitude. The effects at high altitude above 60 km are prominent in L-band and S-band, while the effects at middle and low altitude below 60 km in Ka-band are obvious. The effects in L-band and S-band are much bigger than that in Ka-band and can affect the signal properties of TT&C systems significantly, while the effects in Ka-band are much milder. The waves with large oblique incident angle can encounter much more severe conditions than that with small angle.

EFFECTS OF REENTRY PLASMA FLUCTUATION ON POLARIZATION PROPERTIES OF ELECTROMAGNETIC WAVES

2017-07-18

PIER M

Vol. 58, 159-169, 2017

doi:10.2528/PIERM17021004

download: 337

A GPU Implementation of the Inverse Fast Multipole Method for Multi-Bistatic Imaging Applications

Luis E. Tirado , Galia Ghazi , Yuri Alvarez-Lopez , Fernando Las-Heras and Jose Angel Martinez-Lorenzo

This paper describes a parallel implementation of the Inverse Fast Multipole Method (IFMM) for multi-bistatic imaging configurations. NVIDIAs Compute Unified Device Architecture (CUDA) is used to parallelize and accelerate the imaging algorithm in a Graphics Processing Unit (GPU). The algorithm is validated with synthetic data generated by the Modified Equivalent Current Approximation (MECA) method and experimental data collected by a Frequency-Modulated Continuous Wave (FMCW) radar system operating in the 70-77 GHz frequency band. The presented results show that the IFMM implementation using the CUDA platform is effective at significantly reducing the algorithm computational time, providing a 300X speedup when compared to the single core OpenMP version of the algorithm.

A GPU IMPLEMENTATION OF THE INVERSE FAST MULTIPOLE METHOD FOR MULTI-BISTATIC IMAGING APPLICATIONS

2017-07-17

PIER M

Vol. 58, 147-157, 2017

doi:10.2528/PIERM17051801

download: 395

Design and Measurement of a Novel Seamless Scanning Leaky Wave Antenna in Ridge Gap Waveguide Technology

Xingchao Dong , Hongjian Wang , Fei Xue and Yang Liu

The design and measurement of a novel seamless scanning leaky wave antenna in ridge gap waveguide technology are presented. The impedance matching technique is employed to eliminate the open-stopband (OSB) effect which produces a discontinuity for a seamless scanning leaky wave antenna. Ridge gap waveguide proposed recently is used as the feed structure. The antenna radiates from longitudinal slots of which the leakage constant is designed small to ensure a high directivity. Subsequently, for simplicity, a transition from Ku-band standard waveguide port (WR62) to ridge gap waveguide is designed, which operates within Ku-band with S11 below -15dB. A prototype has been fabricated, and measurements support the simulations obtained by full-wave analysis. The proposed antenna bandwidth is from 12.5GHz to 17.4GHz while seamless scanning is achieved from backward to forward, particularly including broadside radiation. The scanning range is from -9° to 19° with an average gain of 18.3dB.

2017-07-13

PIER M

Vol. 58, 135-145, 2017

doi:10.2528/PIERM17041302

download: 296

Study of Effect of Numerical Breast Phantom Heterogeneity on Dielectric Profile Reconstruction Using Microwave Imaging

Hardik N. Patel and Deepak Ghodgaonkar

Human breast is a heterogeneous medium for microwave signal. Breast cancer detection using microwave imaging is done based on signal scattered by breast tissues at different frequencies. Wave propagation direction is extremely important in heterogeneous medium like human breast. In this paper, the effect of wave propagation direction on the dielectric profile reconstruction is simulated in the presence of noise. X and Y directed transverse electric (TE) waves are considered for numerical breast phantom heterogeneity exploitation. Wave propagating in Y direction results into better dielectric profile reconstruction than X directed wave. Signal to noise ratio is very crucial for microwave imaging because information resides in low power scattered electric signal. Results show that SNR of at least 30 dB is required to detect cancer by solving extremely under-determined system of scattering equations.

2017-07-10

PIER M

Vol. 58, 125-134, 2017

doi:10.2528/PIERM17041401

download: 251

3-d Imaging of High-Speed Moving Space Target via Joint Parametric Sparse Representation

Yuxue Sun , Meng Jiang , Ying Luo , Qun Zhang and Chunhui Chen

The high-speed moving of space targets introduces distortion and migration to range profile, which will have a negative effect on three-dimensional (3-D) imaging of targets. In this paper, based on joint parametric sparse representation, a 3-D imaging method for high-speed moving space target is proposed. First, the impact of high speed on range profile of target is analyzed. Then, based on an L-shaped three-antenna interferometric system, a dynamic joint parametric sparse representation model of echoes from three antennas is established. The dictionary matrix is refined by iterative estimation of velocity. Moreover, an improved orthogonal matching pursuit (OMP) algorithm is proposed to recover interferometric phase information. Finally, with the phase information, interferometric processing is conducted to obtain the 3-D image of target scatterers. The simulation results verify the effectiveness of the proposed method.

3-D IMAGING OF HIGH-SPEED MOVING SPACE TARGET VIA JOINT PARAMETRIC SPARSE REPRESENTATION

2017-07-07

PIER M

Vol. 58, 117-124, 2017

doi:10.2528/PIERM17042702

download: 274

MOEA/d -GO+FDTD for Optimization Design of Fragment-Type Structure

Da-Wei Ding , Xiao-Dong Ding , Jing Xia and Lixia Yang

Fragment-type structure has been used to design antennas and microwave circuits. Special optimization technique, including optimization algorithm and EM software (electromagnetic) simulator, is necessary for the design of this kind of structure. In this paper, a novel optimization technique, MOEA/D-GO+FDTD, is proposed, where MOEA/D-GO (multiobjective evolutionary algorithm combined with enhanced genetic operators) serves as the optimization algorithm and Finite-Difference Time-Domain (FDTD) method serves as the electromagnetic simulator. As an example, a compact bandpass microstrip filter is designed by using MOEA/D-GO+FDTD. Firstly, numerical simulation of the fragment-type microstrip filter by using FDTD method is investigated. Secondly, a microstrip filter operating at 3.8GHz-6.5GHz is designed through optimizing return loss, insertion loss, and out-of-band rejection. Finally, comparison of the computational costs between different electromagnetic simulators verifies high efficiency of the proposed MOEA/D-GO+FDTD.

MOEA/D-GO+FDTD FOR OPTIMIZATION DESIGN OF FRAGMENT-TYPE STRUCTURE

2017-07-06

PIER M

Vol. 58, 107-115, 2017

doi:10.2528/PIERM17052101

download: 278

Backscattering from Electrically Large Target Above Nonlinear Sea Surface

Wei Luo , Yuqi Yang and Honggang Hao

The composite scattering of an electrically large target above nonlinear sea surface is analyzed based on the reciprocity theorem. The two-dimensional nonlinear sea surface is simulated with the Fast Fourier transform (FFT), with which the phase modified two-scale method is utilized to calculate the scattering field of the wind-driven sea surface. The electromagnetic currents of the sea surface, which are excited with plane wave, are calculated with the iterated Kirchhoff approximation (KA).The coupling scattering between the target and the sea surface, which includes the complex scattering matrix of composite scattering, is ingeniously reduced to the integrals involving the target scattering and high order currents of sea surface. A sensitivity analysis is performed for the dependency of the coupling scattering on the target features. The relationship of the full composite scattering model with the sea state is examined, which provides theoretical basis for the target recognition.

BACKSCATTERING FROM ELECTRICALLY LARGE TARGET ABOVE NONLINEAR SEA SURFACE

2017-07-04

PIER M

Vol. 58, 97-106, 2017

doi:10.2528/PIERM17050303

download: 354

A Fourier Phase Mode Approach for Chebyshev Pattern Synthesis in Circular Antenna Array

Mavulluri Ganesh and Konidala Ratna Subhashini

In this article, a novel phase mode analysis for a circular antenna array is discussed. This proposition experiments on the synthesis of Dolph- Chebyshev pattern for circular geometry employing directional element 1+cos(φ). Here, for pattern synthesis a modied uniform sampling method is proposed, and for investigation of continuous current excitation in a circular array, a Fourier phase-mode approach is proposed. The synthesis process permits generation of complex weights for each element to produce the Chebyshev pattern with a desired beamwidth or Side Lobe Level (SLL). The radius is a key factor for a circular geometry and also decides the pattern synthesis, which is determined by using the phase mode concept. Also, this article contributes to the formulation of a mathematical relationship between the number of phase modes (P) and number of antenna elements in the array (N) such as N = 2(P-1).

A FOURIER PHASE MODE APPROACH FOR CHEBYSHEV PATTERN SYNTHESIS IN CIRCULAR ANTENNA ARRAY

2017-07-04

PIER M

Vol. 58, 87-96, 2017

doi:10.2528/PIERM17032403

download: 288

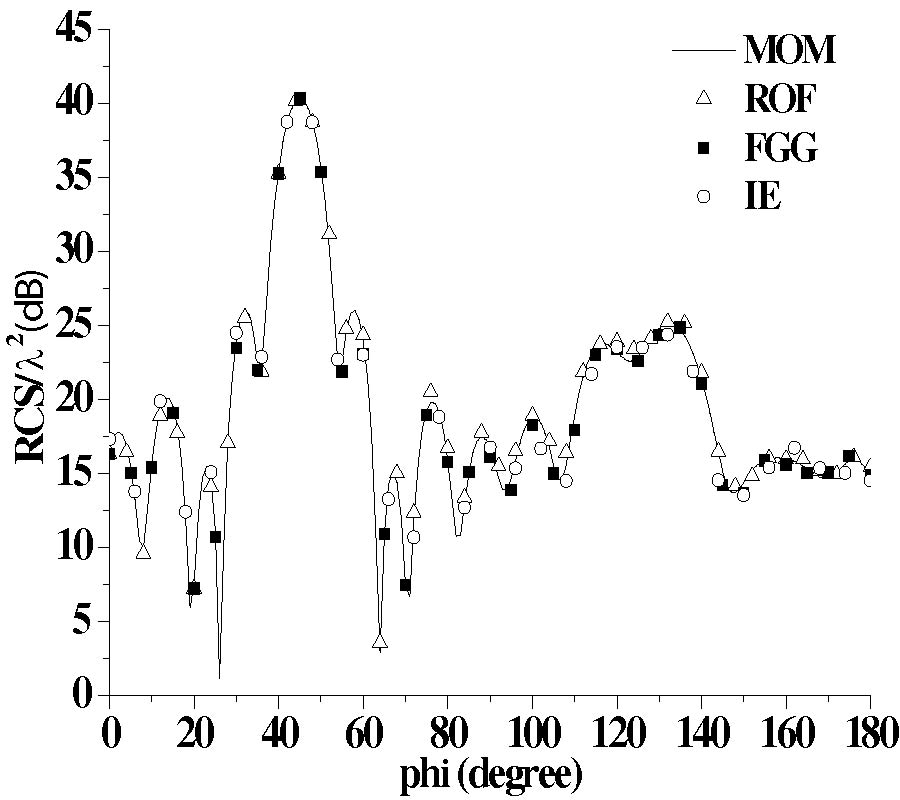
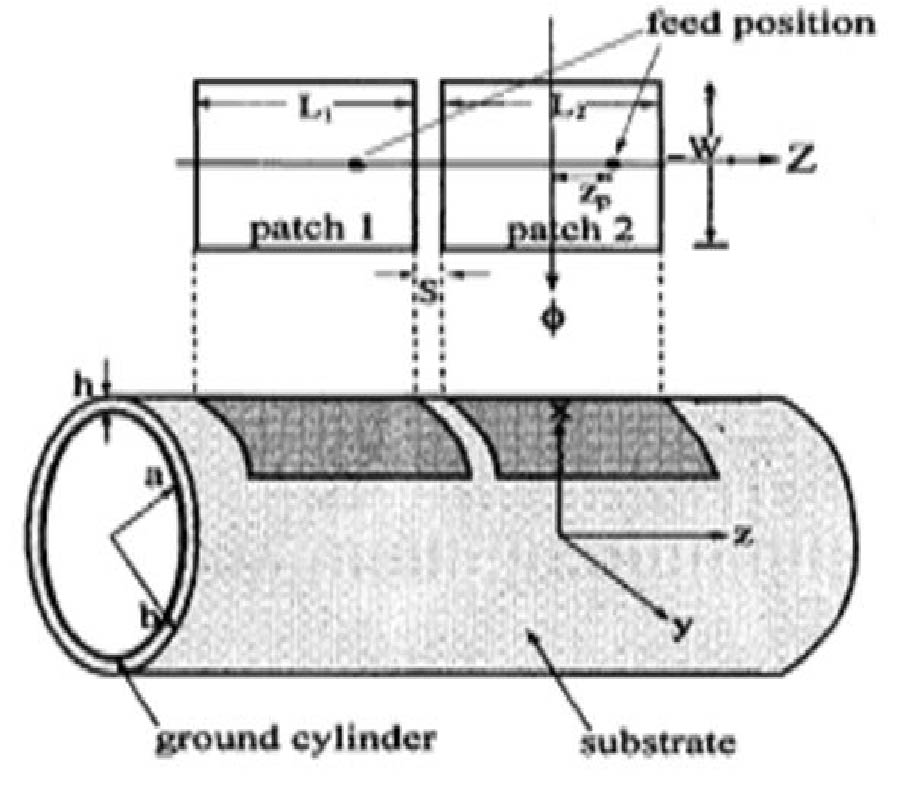
ROF for the Combined Field Integral Equation

Hua-Long Sun , Chuang Ming Tong , Qi Liu and Gao Xiang Zou

This article proposes a computational scheme for a combined field integral equation to compute electromagnetic scattering, which is Reduced order Fitting Green's function's Gradient and Fitting Green's function with Fast Fourier Transform (ROF). This new scheme can greatly reduce computation time compared to integral equation Fast Fourier Transform (IE), as well as Fitting Green's function's Gradient and Fitting Green's function with Fast Fourier Transform (FGG). Firstly, based on the property of Green's functions' integral under special condition, real-coefficient fitting method is utilized to replace the original complex values expression of combination coefficients with the real values. Secondly, a cross-shaped grid named as reduced order grid is presented to reduce computation time for modified near-field coupling impedance. Thirdly, by combining real-coefficient fitting method and reduced order grid, a new scheme of ROF is achieved. Finally, some examples verify ROF, which has advantages, such as higher efficiency than that of IE based on original grid and FGG based on cross-shaped grid, being not sensitive to grid spacing, and keeping the same precision as that of IE based on original grid.

ROF FOR THE COMBINED FIELD INTEGRAL EQUATION

2017-07-04

PIER M

Vol. 58, 73-86, 2017

doi:10.2528/PIERM17030102

download: 282

Modelling Dispersive Behavior of Excitable Cells

Soheil Hashemi and Ali Abdolali

Most of the materials have nearly constant electromagnetic characteristics at low frequencies. Nonetheless, biological tissues are not the same; they are highly dispersive, even at low frequencies. Cable theory is the most famous method for analyzing nerves though a common mistake when studying the model is to consider a constant parameter versus frequency. This issue is discussed in the present article, and the analysis of how to model the dispersion in the cable model is proposed and explained. The proposed dispersive model can predict the behavior of excitable cells versus stimulations with single frequency or wide band signals. In this article, the nondestructive external stimulation by a coil is modeled and computed by finite difference method to survey the dispersion impact. Also, 5% to 80% difference is shown between the results of dispersive and nondispersive models in the 5 Hz to 4 kHz investigation. The disagreement expresses the dispersion notability. The proposed dispersive method assists in accurate device design and signal form optimization. Noise analysis is also achieved by this model, unlike the conventional models, which is essential in the analysis of single neurons or central nervous system, EEG and MEG records.

MODELLING DISPERSIVE BEHAVIOR OF EXCITABLE CELLS

2017-07-04

PIER M

Vol. 58, 65-72, 2017

doi:10.2528/PIERM17050701

download: 543

Design of Nonuniform Metallic Anechoic Chamber for Radiation Pattern Measurement

Ali Farahbakhsh and Mohammad Khalaj-Amirhosseini

Antenna pattern measurement is an essential step in antenna qualification which should be done in anechoic chambers. The common method for anechoic chamber construction is to cover all inside walls by the electromagnetic absorbers. In this paper, a new method is presented to design a fully metallic chamber by controlling the electromagnetic inside the chamber and guiding them to a piece of absorber. Therefore, a desirable quiet zone is formed inside the chamber while a great reduction of absorber usage is achieved. The proposed chamber is analyzed using ray tracing method, and its performance is evaluated by simulation that shows the practicality of the proposed chamber.

DESIGN OF NONUNIFORM METALLIC ANECHOIC CHAMBER FOR RADIATION PATTERN MEASUREMENT

2017-07-02

PIER M

Vol. 58, 57-64, 2017

doi:10.2528/PIERM17051003

download: 373

Principles of Ideal Wideband Reflectarray Antennas

Mohammad Khalaj-Amirhosseini

The principles of ideal wideband RAAs are determined through the idea of distortion-less radiation of a modulated pulse. Two conditions for the cells and one condition for the location of the feed are obtained. The conditions are discussed and clarified by some examples. Each cell requires its own phase at center frequency and its own phase derivative in the desired bandwidth. Some relations are obtained and discussed for the range of required phase derivative of the cells.

PRINCIPLES OF IDEAL WIDEBAND REFLECTARRAY ANTENNAS

2017-06-27

PIER M

Vol. 58, 43-55, 2017

doi:10.2528/PIERM17031601

download: 306

Characteristic Analysis of Phase Glint in InSAR Image Processing

Jing-Ke Zhang , Dahai Dai , Zong-Feng Qi , Yong-Hu Zeng and Liandong Wang

This paper investigates the phase glint problem involved in interferometric synthetic aperture radar (InSAR) image processing, which refers to the multiple scatterer interference of a single pixel, and studies the distribution of interferometric phase in the case of double scatterer interference. It is found that the value range of the observed interferometric phase is related to several factors including the complex scattering coefficient ratio and interferometric phase difference between the elementary scatterers, and no matter what values of interferometric phases of elementary scatterers are taken, the dynamic range of interferometric phase of phase glintis always. This paper also briefly analyzes the impact of phase glint on classical InSAR image processing and man-made target height retrieval, and it is concluded that the phase glint will induce significant height estimating error. Simulation and real data results verify the conclusion.

CHARACTERISTIC ANALYSIS OF PHASE GLINT IN INSAR IMAGE PROCESSING

2017-06-26

PIER M

Vol. 58, 29-41, 2017

doi:10.2528/PIERM17032603

download: 382

Generation and Mitigation of Common-Mode Noise for Differential Traces with Adjacent Ground Line and a Ground Plane

Guang-Hwa Shiue , Zhong-Yan You , Yu-Hsiang Cheng , Ting-Chun Wang , Kuang-Yi Wu , Ming-Yuan Chuang and Chun-Fu Huang

This study investigates the generation and mitigation of common-mode noise (CMN) in a common structure which consists of differential traces with adjacent ground lines and a ground plane. For simplicity, a simple test structure similar to the common structure is proposed. The test structure is divided into three parts. One part is composed of differential traces with adjacent ground lines. The second is composed of differential traces with adjacent ground lines that are connected to a ground plane. The third comprises differential traces with an adjacent ground line and an adjacent ground plane. The generation and mitigation of CMN in these three parts are studied. Test structures with different designs are investigated to confirm the effectiveness of the CMN mitigation schemes. Based on these analyses, design guidelines for mitigating CMN are provided. The proposed design guidelines reduce the peak-to-peak CMN amplitude by 81% from that achieved using unsuitable design of test structure. In the frequency domain, the reduction of the magnitude of differential-to-common mode conversion (|S_cd21|) at the resonant peaks exceeded 40 dB in the frequency range 0 GHz~6 GHz. Finally, a favorable comparison between simulated and measured results verifies the favorable CMN mitigation performance of the proposed design guidelines.

GENERATION AND MITIGATION OF COMMON-MODE NOISE FOR DIFFERENTIAL TRACES WITH ADJACENT GROUND LINE AND A GROUND PLANE

2017-06-23

PIER M

Vol. 58, 21-27, 2017

doi:10.2528/PIERM17041705

download: 339

Electromagnetic Retarded Potential Induced by Quantum Vacuum Polarization

Leng Liao , Ruiqiang Zhao , Hong Zhang , Jianting Zhou and Yewang Bie

Based on order one-loop effective Lagrangian derived from the 2-point photon vertex in quantum electrodynamics, we obtain a quantum modified Maxwell equations, and the classical expression of retarded potential is consequently modified by these equations. The results indicate that, due to the time-space non-locality of vacuum polarization, the vacuum polarization current is delayed relative to the field variation and induces a series of additional retarded potentials except for the classical part of retarded potential. Particularly, compared to the classical potential, these additional potentials are further retarded. Because the retard potential is the base of theory of electromagnetic radiation, the results of this work are of great value to the studies of quantum effect in ultra-intense electromagnetic radiation.

2017-06-21

PIER M

Vol. 58, 11-19, 2017

doi:10.2528/PIERM17022012

download: 322

Effect of Varying Curvature and Inter Element Spacing on Dielectric Coated Conformal Microstrip Antenna Array

Prasanna Kumar Singh and Jasmine Saini

In the design of a conformal patch antenna array, a special care must be taken regarding the placement of elements and curvature bending. Presently, the authors try to explain the effect of these two factors on the key parameters such as return loss, mutual coupling, gain and directivity. Here, the analyses of parameters are done under the consideration of dielectric coated two-element antenna array model. This paper attempts to examine the characteristics of the dielectric coated conformal antenna array by varying its inter element spacing on the changing cylindrical geometry. The two-element conformal array is considered in E-plane and H-plane configurations, and its parameters are analyzed using full wave analysis and verified by HFSS tool. A comparative study shows that the E-plane configuration gives better result than H-plane configuration.

EFFECT OF VARYING CURVATURE AND INTER ELEMENT SPACING ON DIELECTRIC COATED CONFORMAL MICROSTRIP ANTENNA ARRAY

2017-06-20

PIER M

Vol. 58, 1-10, 2017

doi:10.2528/PIERM17020904

download: 246

Fast and Accurate Determination of the Complex Resonant Frequency of a Multilayer Circular Cavity Using Chebyshev's Root-Finder

David Marques-Villarroya , Felipe Penaranda-Foix , Beatriz Garcia-Banos , Jose Manuel Cataia-Civera and Jose Daniel Gutierrez-Cano

In this paper, a general multilayer circular cavity with N slabs is analyzed analytically, obtaining characteristic equations for TE and TM modes to compute the complex resonant frequency efficiently using an algorithm based on Chebyshev's root finder. The accuracy of the solutions is compared with full-wave circuit method, and the computational speed to achieve the roots of the characteristic equations is also compared with Cauchy Integral Method, which is commonly used to obtain complex roots. Furthermore, the relationship between the amplitudes of the different regions is obtained, whereby the whole structure can be analyzed as a single one from now on.