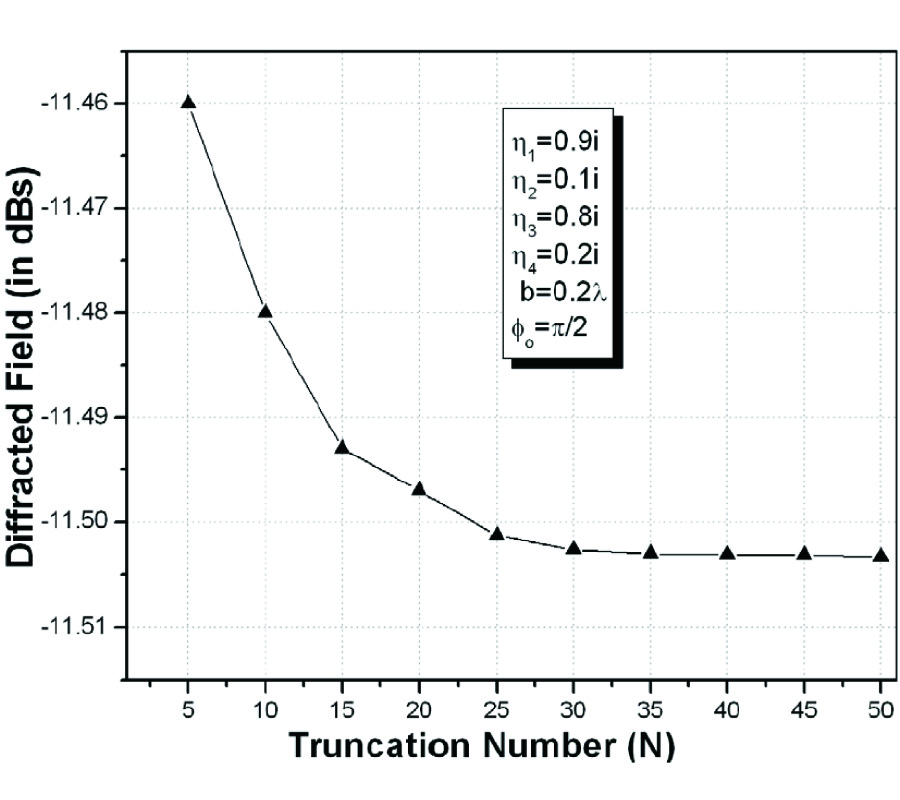
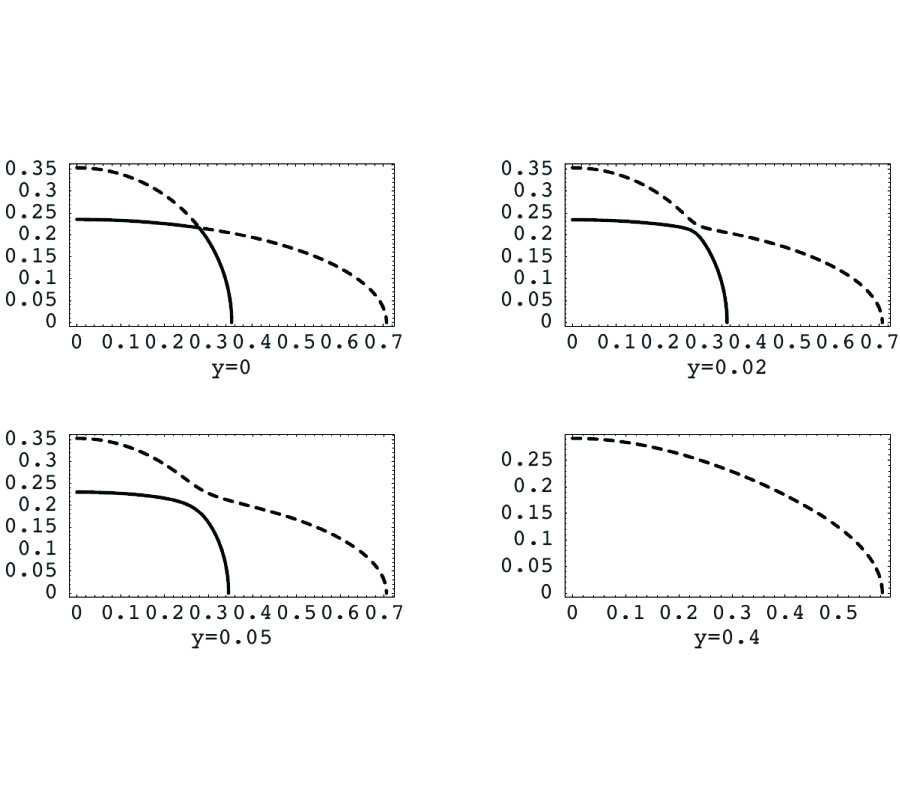
Matias Dahl
A Gaussian beam is an asymptotic solution to Maxwell's equations that propagate along a curve; at each time instant its energy is concentrated around one point on the curve. Such a solution is of the form
E = Re{eiPθ(x,t)E0(x, t)}, where
E0 is a complex vector field, P >0 is a big constant, and θ is a complex second order polynomial in coordinates adapted to the curve. In recent work by A. P. Kachalov, electromagnetic Gaussian beams have been studied in a geometric setting. Under suitable conditions on the media, a Gaussian beam is determined by Riemann-Finsler geometry depending only on the media. For example, geodesics are admissible curves for Gaussian beams and a curvature equation determines the second order terms in θ. This work begins with a derivation of the geometric equations for Gaussian beams following the work of A. P. Kachalov. The novel feature of this work is that we characterize a class of inhomogeneous anisotropic media where the induced geometry is Riemannian. Namely, if ε, μ are simultaneously diagonalizable with eigenvalues ε
i, μ
j , the induced geometry is Riemannian if and only if ε
iμ
j = ε
jμ
i for some
i ≠ j. What is more, if the latter condition is not met, the geometry is ill-behaved. It is neither smooth nor convex. We also calculate Riemannian metrics for different media. In isotropic media,
gij = εμδ
ij and in more complicated media there are two Riemannian metrics due to different polarizations.