Parabolic Equation Solution of Tropospheric Wave Propagation Using FEM
Stergios Isaakidis and
Thomas Xenos
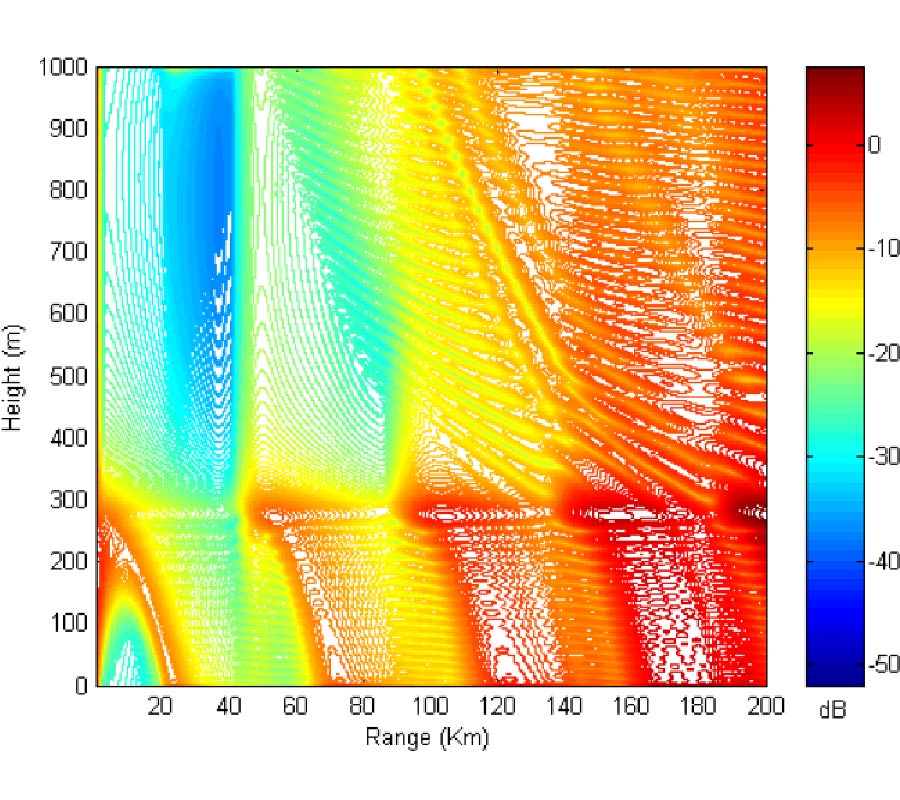
Abstract-In this work,the parabolic equation applied on radiowave and microwave tropospheric propagation, properly manipulated, and resulting in a one-dimensional form, is solved using the Finite Element Method (FEM). The necessary vertical tropospheric profile characteristics are assigned to each mesh element, while the solution advances in small and constant range segments, each excited by the solution of the previous step. This is leading to a marching algorithm, similar to the widely used Split Step formulation. The surface boundary conditions including the wave polarization and surface conductivity properties are directly applied to the FEM system of equations. Since the FEM system returns the total solution, a technique for the separation of the transmitted and reflected waves is also presented. This method is based on the application of the Discrete Fourier Transform (DFT) in the space domain, which allows for the separation of the existing wave components. Finally, abnormal tropospheric condition propagation is being employed to assess the method, while the results are compared to those obtained using the Advance Refractive Prediction System (AREPS v.3.03) software package.