PIER | PIER Journals

2014-11-17

PIER

Vol. 149, 281-289, 2014

download: 200

Realizing Flexible Ultra-Flat-Band Slow Light in Hybrid Photonic Crystal Waveguides for Efficient Out-of-Plane Coupling

Jianhao Zhang , Yaocheng Shi and Sailing He

The realization of slow light with ultra-flat dispersion in hybrid photonic crystal (HPhC) waveguide is systematically investigated. Metal strips have been introduced to the photonic crystal (PhC) waveguide. The dispersion of the odd mode is commendably flattened in the leaky region. Ultra-flat-band slow light with nearly constant average group indices of 192 over 2 nm (i.e., 330 GHz) bandwidth is achieved. Flexible tuning for the ultra-high group index can also be achieved while keeping the normalized delay-bandwidth product fairly high. The introduction of the metal strips is further demonstrated to help reduce the azimuthal angle of the farfield and provide a high coupling efficiency.

REALIZING FLEXIBLE ULTRA-FLAT-BAND SLOW LIGHT IN HYBRID PHOTONIC CRYSTAL WAVEGUIDES FOR EFFICIENT OUT-OF-PLANE COUPLING

2014-11-17

PIER

Vol. 149, 275-280, 2014

doi:10.2528/PIER14102112

download: 221

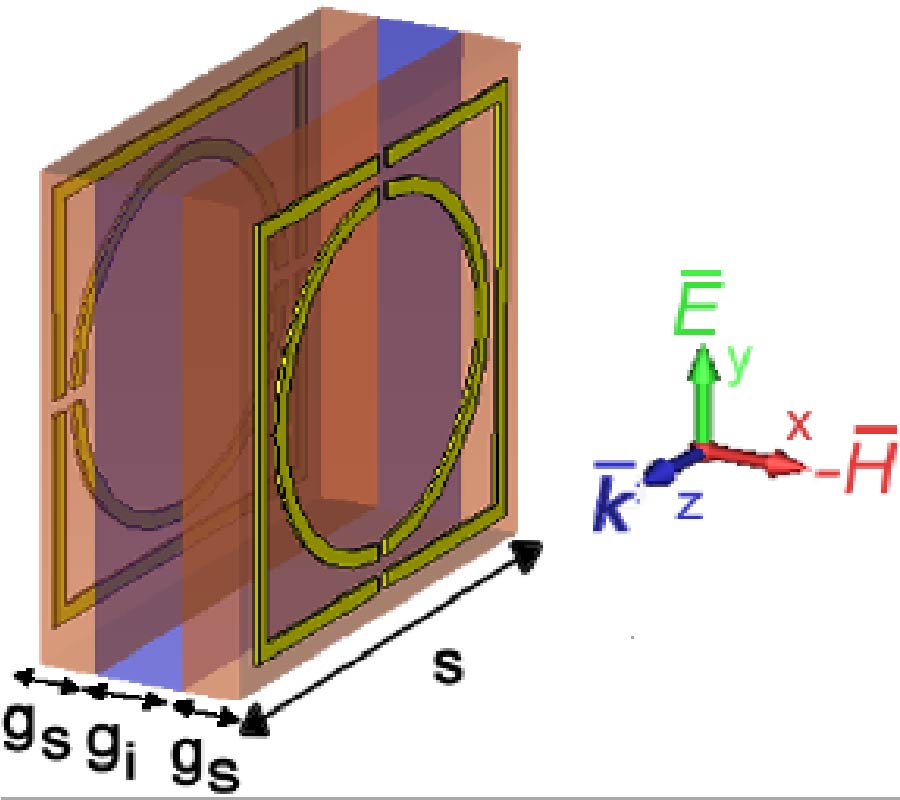
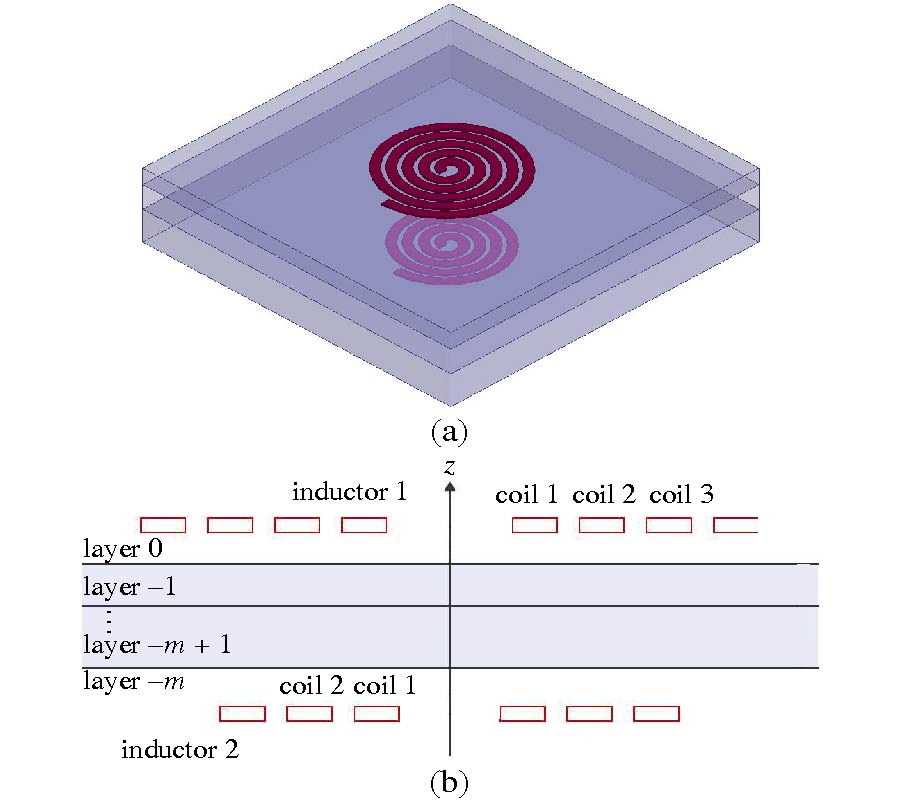
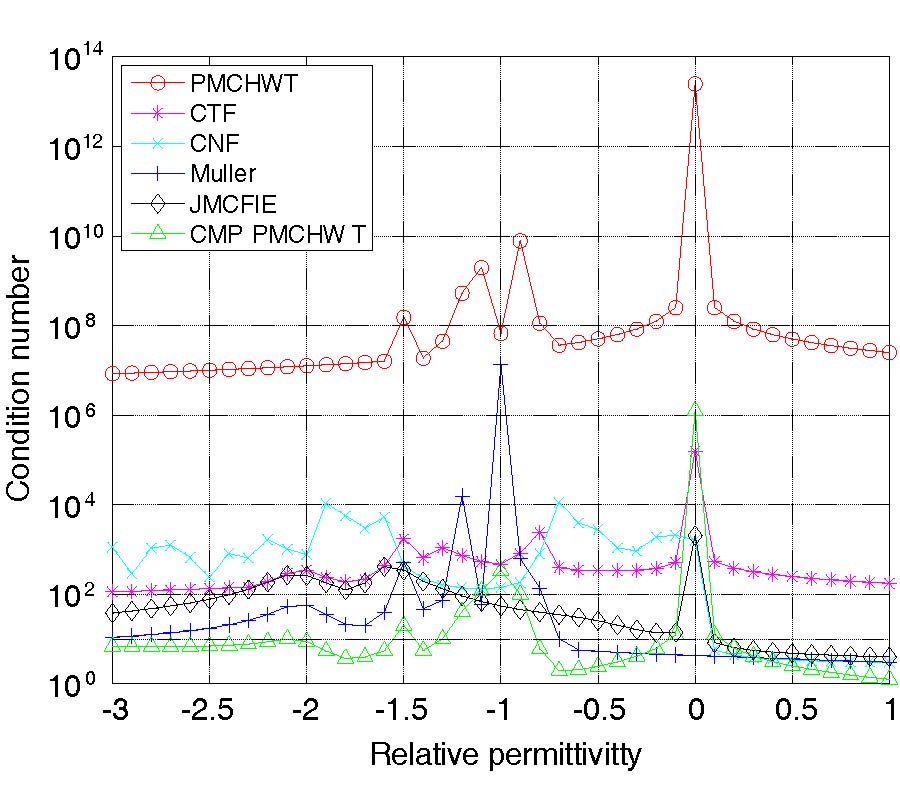
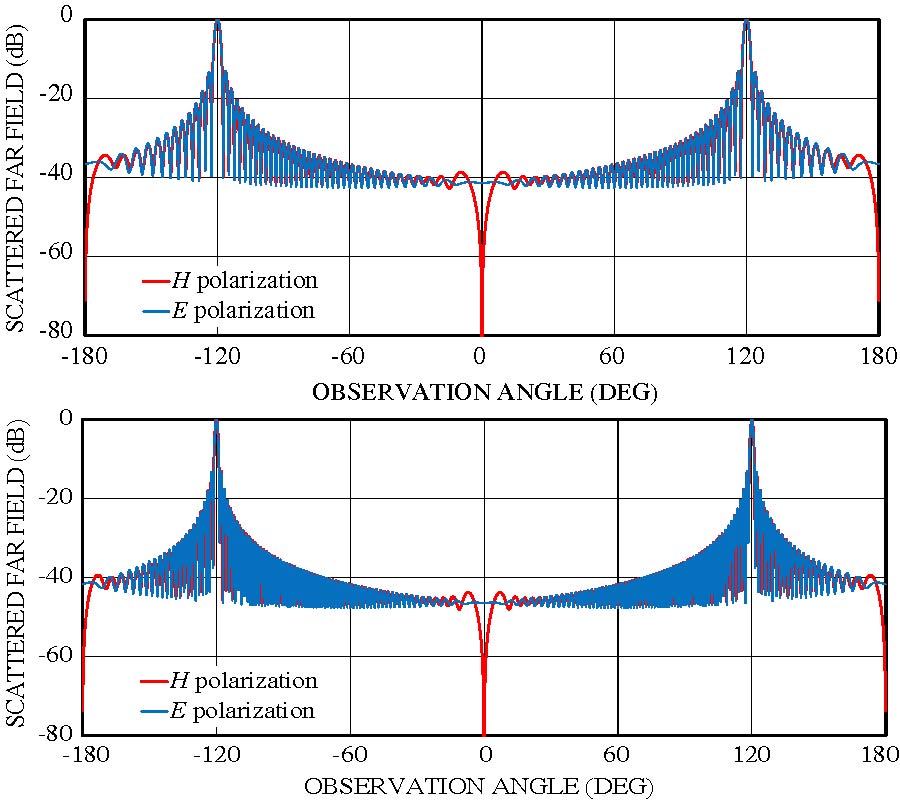
Casimir Force for Complex Objects Using Domain Decomposition Techniques

Phillip R. Atkins , Weng Cho Chew , Maokun Li , Lin E. Sun , Zu-Hui Ma and Li Jun Jiang

A method for calculating the Casimir force between large, complex 3D objects is presented. Difficulties have previously arisen in broadband multiscale calculation using CEM methods. To expand the range of problems that can be calculated, we use an integral equation, domain decomposition method (DDM) and argument principle to derive the Casimir force formula. The broadband integral equation DDM, which is the augmented equivalence principle algorithm (A-EPA), allows for an efficient broadband solution of large, complex objects. A-EPA subdivides a complex problem into separate smaller subproblems that are later recombined into a reduced matrix. This yields a reduced number of unknowns for complex structures making them feasible with modest computer resources. We demonstrate the advantages of the A-EPA by simulating large, finite, 3D, unaligned corrugated plates, which have previously only been modeled approximately as infinite plates using 2D techniques.

CASIMIR FORCE FOR COMPLEX OBJECTS USING DOMAIN DECOMPOSITION TECHNIQUES

2014-11-10

PIER

Vol. 149, 251-273, 2014

doi:10.2528/PIER14102506

download: 338

Transformation Optics and Applications in Microwave Frequencies (Invited Paper)

Wei Xiang Jiang , Wen Xuan Tang and Tie-Jun Cui

Modern electrical and communication technologies benefit from classical electrodynamics and electric circuits, both of which are based on the Maxwell's equations. Using the property of metric invariance in Maxwell's Equations, transformation optics has been proposed and achieves a rapid progress in the past decade. Transformation optics is a method for the conceptual design of complex electromagnetic media, offering opportunities for the control of electromagnetic waves. In this paper, we introduce the general theory of transformation optics and discuss the recent development on the transformation devices in the microwave band, such as non-singular invisibility cloak and its realization in dc circuit, three-dimensional ground-plane cloaks, flattened Luneburg lens, high-performance antennas, and high-resolution imaging lens. Some of the transformation-optics-based devices are expected to have further impact on the microwave engineering applications.

TRANSFORMATION OPTICS AND APPLICATIONS IN MICROWAVE FREQUENCIES (Invited Paper)

2014-11-09

PIER

Vol. 149, 217-249, 2014

doi:10.2528/PIER14092503

download: 496

Reflections on Maxwell's Treatise (Invited Paper)

Arthur D. Yaghjian

Over a period of about twenty years, Maxwell's determination and unification of the equations of electricity and magnetism evolved from his first paper on the subject in 1855-56, ``Faraday's Lines of Force,'' to the publication of his Treatise on Electricity and Magnetism in 1873. Notwithstanding the many historical accounts and textbooks devoted to Maxwell's work, I have not been able to find a reasonably concise, yet definitive summary of the fundamentals of exactly what Maxwell did in his Treatise and how he did it. This paper represents my own attempt to provide such a summary.

2014-11-04

PIER

Vol. 149, 205-216, 2014

doi:10.2528/PIER14100505

download: 239

Metasurface Synthesis for Time-Harmonic Waves: Exact Spectral and Spatial Methods (Invited Paper)

Mohamed A. Salem , Karim Achouri and Christophe Caloz

Two exact approaches to synthesize metasurfaces for time-harmonic waves are discussed. The first approach is a spectral approach based on wave momentum conservation. Here, the spectral approach is applied to scalar and paraxial wave transformations. This approach effectively allows the arbitrary translation of the transformation plane parallel to the metasurface. The second approach is a direct-space approach based on the extraction of the susceptibility tensors of the metasurface elements. This approach is applied to vectorial field transformation and can be used for single or multiple transformations. An example of wave transformation by a metasurface is illustrated for each of the two approaches.

METASURFACE SYNTHESIS FOR TIME-HARMONIC WAVES: EXACT SPECTRAL AND SPATIAL METHODS (Invited Paper)

2014-11-03

PIER

Vol. 149, 193-204, 2014

doi:10.2528/PIER14053108

download: 333

Electromagnetic Analysis of an Aperture Modified TEM Cell Including an ITO Layer for Real-Time Observation of Biological Cells Exposed to Microwaves

Malak Soueid , Sophie Kohler , Lynn Carr , Sylvia M. Bardet , Rodney P. O'Connor , Philippe Leveque and Delia Arnaud-Cormos

We propose to analyze the aperture and ITO layer presence of a modified transverse electromagnetic (TEM) cell. This TEM cell can be used to study the potential effects of microwave electromagnetic fields on biological cells. This modified delivery device allows realtime observation of biological cells during exposure. Microscopic observation is achieved through an aperture in the lower wall of the TEM cell that is sealed with a 700-nm film of the transparent conducting material Indium tin oxide (ITO). To determine the device efficiency, numerical and experimental electromagnetic dosimetry was conducted. For assessing the effect of the aperture on the specific absorption rate (SAR) in the exposed sample, a plastic Petri dish containing cell culture medium, full-wave 3-D electromagnetic simulations and temperature measurements were performed. For 1-W input power, the SAR values obtained at 1.8 GHz in the sample exposed in the TEM cell with the sealed or non-sealed aperture of 20-mm diameter were 1.1 W/kg and 23.6 W/kg, respectively. An excellent homogeneity of the SAR distribution was achieved when the aperture was sealed with the ITO layer. The performance of the delivery system was confirmed by microwave exposure and simultaneous observation of living cells.

ELECTROMAGNETIC ANALYSIS OF AN APERTURE MODIFIED TEM CELL INCLUDING AN ITO LAYER FOR REAL-TIME OBSERVATION OF BIOLOGICAL CELLS EXPOSED TO MICROWAVES

2014-10-31

PIER

Vol. 149, 183-192, 2014

doi:10.2528/PIER14063002

download: 239

Compact Waveguided Metamaterials for Suppression of Mutual Coupling in Microstrip Array

Zeeshan Qamar and Hyun Chang Park

In this paper, suppression of mutual coupling is achieved using compact waveguided metamaterials in between elements of a densely packed microstrip array. Both the E-plane internally folded complementary split ring resonator and the H-plane internally folded complementary split ring resonator are employed to reduce the mutual coupling between adjacent elements. Coupling suppression of 18 dB and 9 dB, for elements in the E-plane and H-plane, respectively, is demonstrated. Due to the compact size of the waveguided metamaterials, the edge-to-edge separation between elements is kept at only 0.093λ₀. With the same elements spacing, a 2×2 array is also simulated with compact WG-MTMs. The proposed structure reduces the array size and enables the implementation of compact Multiple-Input-Multiple-Output systems.

COMPACT WAVEGUIDED METAMATERIALS FOR SUPPRESSION OF MUTUAL COUPLING IN MICROSTRIP ARRAY

2014-10-15

PIER

Vol. 149, 173-182, 2014

doi:10.2528/PIER14100303

download: 263

A Third Way to Cloak an Object: Cover-Up with a Background Object (Invited Paper)

Fei Sun and Sailing He

Based on a space folding transformation, we propose a new way to hide an object in full space,namely, to cover-up the scattering of the hidden object with the scattering of a background object so that only the scattering of the background object can be detected by an outside observer and the hidden object disappears electromagnetically (a very weak ``ghost image'' or perturbation may appear inside the strong background object image in an experiment). The present method is essentially different from previous methods of cloaking an object. This work furthers efforts to achieve invisibility and conceal an object in a real environment in full space.

A THIRD WAY TO CLOAK AN OBJECT: COVER-UP WITH A BACKGROUND OBJECT (Invited Paper)

2014-10-14

PIER

Vol. 149, 161-171, 2014

doi:10.2528/PIER14080606

download: 303

Breast Imaging Using Microwave Tomography with Radar-Based Tissue-Regions Estimation

Anastasia Baran , Douglas J. Kurrant , Amer Zakaria , Elise C. Fear and Joe LoVetri

Microwave tomography (MWT) and a radar-based region estimation technique are combined to create a novel algorithm for biomedical imaging with a focus on breast cancer detection and monitoring. The region estimation approach is used to generate a patient-specific spatial map of the breast anatomy that includes skin, adipose and fibroglandular regions, as well as their average dielectric properties. This map is incorporated as a numerical inhomogeneous background into an MWT algorithm based on the finite element contrast source inversion (FEM-CSI) method. The combined approach reconstructs finer structural details of the breast and better estimates the dielectric properties than either technique used separately. Numerical results obtained with the novel combined algorithmic approach, based on synthetically generated breast phantoms, show significant improvement in image quality.

BREAST IMAGING USING MICROWAVE TOMOGRAPHY WITH RADAR-BASED TISSUE-REGIONS ESTIMATION

2014-10-14

PIER

Vol. 149, 147-160, 2014

doi:10.2528/PIER14073103

download: 234

A Design of Multiband, Dual-Polarization, Beam-Switchable Dual-Antenna for Indoor Base Stations

Viet-Anh Nguyen , Rao Shahid Aziz , Seong-Ook Park and Giwan Yoon

A novel multiband, dual-polarization, beam-switched dual-antenna design, covering some LTE and WLAN bands, has been proposed for indoor base stations. The proposed design consists mainly of dual modified monopole antennas. The horizontal antenna consists of four printed monopole elements, and the control circuit using p-i-n diodes has been implemented for feeding to each monopole element. The vertical monopole antenna beam patterns are controlled by reconfigurable frequency selective reflectors (RFSR) technique. The p-i-n diodes have been utilized for switching mechanism to feed the four RFSR. The measured and simulated results indicate that the antenna system possesses multiband and dual polarization. It has been observed from the simulated and measured reflection coefficients |S₁₁| that the presented dual antenna system supports both LTE (1.7-2.1 GHz) and WLAN (2.5 GHz and 5.8 GHz) band frequencies. Moreover, the radiation characteristics show dual-polarization behaviors of the presented antenna system and beam switching states suitable for small cell indoor-base stations.

A DESIGN OF MULTIBAND, DUAL-POLARIZATION, BEAM-SWITCHABLE DUAL-ANTENNA FOR INDOOR BASE STATIONS

2014-10-13

PIER

Vol. 149, 133-145, 2014

doi:10.2528/PIER14072502

download: 997

A Satellite Multiple-Beam Antenna for High-Rate Data Relays

Lukasz A. Greda , Andreas Winterstein , Achim Dreher , Sascha A. Figur , Bernhard Schonlinner , Volker Ziegler , Marco Haubold and Martin W. Brueck

This paper describes the concept and the development of key components of a novel multiple-beam antenna for satellite applications. The antenna is designed to be used in a transparent high-rate data relay system that links several low earth orbit (LEO) satellites to a single ground station via a satellite positioned on a geostationary orbit (GEO). The proposed antenna is based on the concept of an array-fed reflector. The antenna can track LEO satellites by switching between different subarrays of a bigger multifeed array using a reconfigurable switch matrix based on radio frequency micro-electro-mechanical system (RF MEMS) switches. The radiation characteristic of the antenna is further improved by combining digital beamforming with beam switching. In order to validate the proposed antenna concept and to show its suitability for space applications a demonstrator has been built. Measurements of the antenna's key components and of the demonstration system are given.

A SATELLITE MULTIPLE-BEAM ANTENNA FOR HIGH-RATE DATA RELAYS

2014-09-30

PIER

Vol. 149, 119-132, 2014

doi:10.2528/PIER14080503

download: 258

Time-Domain Microwave Radar Applied to Breast Imaging: Measurement Reliability in a Clinical Setting

Emily Porter , Adam Santorelli and Milica Popovic

This work presents an evaluation of the measurement challenges in clinical testing of our microwave breast cancer screening system. The time-domain radar system contains a multistatic 16-antenna hemi-spherical array operating in the 2-4 GHz frequency range. We investigate, for the first time with such a system in clinical trials, the repeatability of measurements and its effect on image reconstruction. We record vertical and horizontal measurement uncertainties under different scenarios and verify, using previously introduced compensation methods, that they can be successfully reduced to an acceptable level from the standpoint of image reconstruction. We also examine how placement of an immersion medium can affect collected breast scan data. Finally, we probe the repeatability and consistency of measurements with patients. With the goal of confirming the feasibility of frequent breast health monitoring, with our system, we obtain a total of 342 breast scans collected over 57 patient visits to determine how much scan data varies when there are no changes in between scans, and how much it varies when the patient is repositioned in the system. We confirm that, by taking care in patient positioning in the system and with respect to the immersion medium, the measurement repeatability is high.

TIME-DOMAIN MICROWAVE RADAR APPLIED TO BREAST IMAGING: MEASUREMENT RELIABILITY IN A CLINICAL SETTING

2014-09-26

PIER

Vol. 149, 109-118, 2014

doi:10.2528/PIER14070706

download: 301

Magnetic Field Induced by Wake of Moving Body in Wind Waves (Invited Paper)

Xiaojian Zhu and Mingyao Xia

A general procedure to evaluate the electromagnetic fields generated by moving seawater through the geomagnetic field is proposed. It contains two essential steps: modeling of velocity vector of seawater according to its dynamic mechanism, and solution of Maxwell equations under a stratified ocean configuration. Two kinds of motions are considered in this work, wind-driven waves and wakes due to a moving body. The ocean is taken to be infinitely deep at the moment. Both the velocity vector and magnetic field are expressed as a superposition of sinusoidal waves. Simulation results show that the magnetic fields produced by moderate wind waves or a middle-size body moving at moderate speed are on the order of one hundred pico-Tesla near the sea level. The spectrum characteristics of the two kind magnetic anomalies are distinct.

MAGNETIC FIELD INDUCED BY WAKE OF MOVING BODY IN WIND WAVES (Invited Paper)

2014-09-07

PIER

Vol. 149, 101-108, 2014

doi:10.2528/PIER14070306

download: 205

On Inhomogeneous Metamaterials Media: a New Alternative Method for Analysis of Electromagnetic Fields Propagation

Consuelo Bellver-Cebreros and Marcelo Rodriguez-Danta

The analysis of waves propagation in homogeneous anisotropic media constitutes a classical topic in every field of science and has been preferentially discussed using locally plane waves. Specific physical quantities and their behaviour laws are really what make the difference. Although the use of Fourier transform enables an approach formally analogous to that of plane waves in linear evolution equations, its application to constitutive equations of inhomogeneous media involves cumbersome convolution products that mask the solution. This paper proposes a polar representation (amplitude and phase) of electromagnetic fields, that appears to be more suitable and provides two sets of equations that can be easily decoupled, reducing the problem to the superposition of two simpler ones. The procedure is based upon the following steps: a) The identification of dispersion equation with Hamilton-Jacobi equation yields the evolution laws of rays and/or wave-fronts. b) From the knowledge of tensor ε(r) at any point r of the wave front (or the ray), the use of the intrinsic character (conjugation relations) of fields, introduced by the authors in a previous work, together with ray velocity or phase gradient (found in the first step) the remaining fields are immediately obtained.

ON INHOMOGENEOUS METAMATERIALS MEDIA: A NEW ALTERNATIVE METHOD FOR ANALYSIS OF ELECTROMAGNETIC FIELDS PROPAGATION

2014-09-06

PIER

Vol. 149, 85-99, 2014

doi:10.2528/PIER14070210

download: 267

Influence of Active Nano Particle Size and Material Composition on Multiple Quantum Emitter Enhancements: Their Enhancement and Jamming Effects (Invited Paper)

Samel Arslanagic and Richard Ziolkowski

In the 150 years that scientists and engineers have used Maxwell's equations to describe electromagnetic phenomena, canonical scattering and radiating problems have played a very important role, providing explanations of and insights into their underlying physics. With the same intent, a variety of active coated nano-particles are examined here theoretically with regard to their ability to effectively enhance or jam(cloak) the responses of quantum emitters, e.g., fluorescing molecules, and nano-antennas to an observer located in their far-field regions. The investigated spherical particles consist of a gain-impregnated silica nano-core covered with a nano-shell of a specific plasmonic material. Attention is devoted to the influence of the over-all size of these particles and their material composition on the obtained levels of active enhancement or jamming. Silver, gold and copper are employed as their nano-shells. The over-all diameters of the investigated coated nano-particles are taken to be 20 nm, 40 nm, and 60 nm, while maintaining the same ratio of the core radius and shell thickness. It is shown that the jamming levels, particularly when several emitters are present, are significantly larger for particles of larger sizes. These configurations are also shown to lead to the largest enhancement levels of the surrounding quantum emitters. Furthermore, for a fixed particle size and for a gain constant that produces the largest enhancement peak at optical wavelengths, it is demonstrated that these larger levels are most notable when the nano-shell is gold.

INFLUENCE OF ACTIVE NANO PARTICLE SIZE AND MATERIAL COMPOSITION ON MULTIPLE QUANTUM EMITTER ENHANCEMENTS: THEIR ENHANCEMENT AND JAMMING EFFECTS (Invited Paper)

2014-09-02

PIER

Vol. 149, 69-84, 2014

doi:10.2528/PIER14060904

download: 1316

Vector Potential Electromagnetics with Generalized Gauge for Inhomogeneous Media: Formulation (Invited Paper)

Weng Cho Chew

Vector and scalar potential formulation is valid from quantum theory to classical electromagnetics. The rapid development in quantum optics calls for electromagnetic solutions that straddle quantum physics as well as classical physics. The vector potential formulation is a good candidate to bridge these two regimes. Hence, there is a need to generalize this formulation to inhomogeneous media. A generalized gauge is suggested for solving electromagnetic problems in inhomogenous media which can be extended to the anistropic case. The advantages of the resulting equations are their absence of low-frequency catastrophe. Hence, usual differentialequation solvers can be used to solve them over multi-scale and broad bandwidth. It is shown that the interface boundary conditions from the resulting equations reduce to those of classical Maxwell's equations. Also, classical Green's theorem can be extended to such a formulation, resulting in similar extinction theorem, and surface integral equation formulation for surface scatterers. The integral equations also do not exhibit low-frequency catastrophe as well as frequency imbalance as observed in the classical formulation using E-H fields. The matrix representation of the integral equation for a PEC scatterer is given.

VECTOR POTENTIAL ELECTROMAGNETICS WITH GENERALIZED GAUGE FOR INHOMOGENEOUS MEDIA: FORMULATION (Invited Paper)

2014-08-28

PIER

Vol. 149, 55-67, 2014

doi:10.2528/PIER14070111

download: 438

Chiral Metamaterial Based Multifunctional Sensor Applications

Muharrem Karaaslan and Mehmet Bakir

In this work, sensor abilities of a chiral metamaterial based on split ring resonators with double splits (SRDS) are demonstrated both theoretically and experimentally in X band range. This study is based on transmission measurements and simulations monitoring the resonance frequency changes with respect to the thickness of the sensing layer and permittivity values. Experimental and simulated results show that the resonance frequency of the chiral metamaterial based SRDS sensor is linearly related to permittivity and the thickness of the sensor layer which creates a suitable approach for sensing environment and organic parameters. When the sensor layer filled with the related material, changes in the tissue temperature, sand humidity and calcium chloride density lead to resonance frequency changes. The physical mechanisms are explained by using both equivalent circuit model and the fundamental sensitivity theorem of chiral sensors. This is the first study as a sensing mechanism based on the chiral metamaterials in X band range.

CHIRAL METAMATERIAL BASED MULTIFUNCTIONAL SENSOR APPLICATIONS

2014-08-26

PIER

Vol. 149, 45-54, 2014

doi:10.2528/PIER14072404

download: 243

Full-Wave Semi-Analytical Modeling of Planar Spiral Inductors in Layered Media

Yan Lin Li and Sheng Sun

In this paper, we present a full-wave semi-analytical solution to calculate the self and mutual impedances of two coupled spiral inductors with rectangular cross sections. In low-frequency electromagnetism, the self and mutual impedance of planar spiral inductors can be obtained based on the eddy current approximation, where the displacement current is disregarded. As the frequency increases, the size of the system can be designed to be smaller. However, the displacement current becomes more important in inductively-coupled systems. By directly deriving the Maxwell's equations without the eddy current assumption, the obtained full-wave model could be applied to both homogeneous and planarly layered media for wireless power transfer systems. Compared to the traditional methods, the newly derived impedances show a considerable discrepancy at GHz frequencies for millimeter-sized inductors, indicating the significance of the displacement current if the operating frequency of wireless power transmission reaches the GHz-range.

FULL-WAVE SEMI-ANALYTICAL MODELING OF PLANAR SPIRAL INDUCTORS IN LAYERED MEDIA

2014-08-23

PIER

Vol. 149, 15-44, 2014

doi:10.2528/PIER14070105

download: 744

Surface and Volume Integral Equation Methods for Time-Harmonic Solutions of Maxwell's Equations (Invited Paper)

Pasi Yla-Oijala , Johannes Markkanen , Seppo Jarvenpaa and Sami P. Kiminki

During the last two-three decades the importance of computer simulations based on numerical full-wave solutions of Maxwell's has continuously increased in electrical engineering. Software products based on integral equation methods have an unquestionable importance in the frequency domain electromagnetic analysis and design of open-region problems. This paper deals with the surface and volume integral equation methods for finding time-harmonic solutions of Maxwell's equations. First a review of classical integral equation representations and formulations is given. Thereafter we briefly overview the mathematical background of integral operators and equations and their discretization with the method of moments. The main focus is on advanced techniques that would enable accurate, stable, and scalable solutions on a wide range of material parameters, frequencies and applications. Finally, future perspectives of the integral equation methods for solving Maxwell's equations are discussed.

SURFACE AND VOLUME INTEGRAL EQUATION METHODS FOR TIME-HARMONIC SOLUTIONS OF MAXWELL'S EQUATIONS (Invited Paper)

2014-08-23

PIER

Vol. 149, 1-13, 2014

doi:10.2528/PIER14063007

download: 318

Wiener-Hopf Analysis of the h -Polarized Plane Wave Diffraction by a Finite Sinusoidal Grating (Invited Paper)

Toru Eizawa and Kazuya Kobayashi

The diffraction by a finite sinusoidal grating is analyzed for the H-polarized plane wave incidence using the Wiener-Hopf technique combined with the perturbation method. Assuming the depth of the grating to be small compared with the wavelength and approximating the boundary condition on the grating surface, the problem is reduced to the diffraction problem involving a flat strip with a certain mixed boundary condition. Introducing the Fourier transform for the unknown scattered field and applying an approximate boundary condition together with a perturbation series expansion for the scattered field, the problem is formulated in terms of the zero-order and first-order Wiener-Hopf equations. The Wiener-Hopf equations are solved via the factorization and decomposition procedure leading to the exact and asymptotic solutions. Taking the inverse Fourier transform and applying the saddle point method, the scattered field expression is explicitly derived. Scattering characteristics of the grating are discussed in detail via numerical examples of the far field intensity.